Abstract
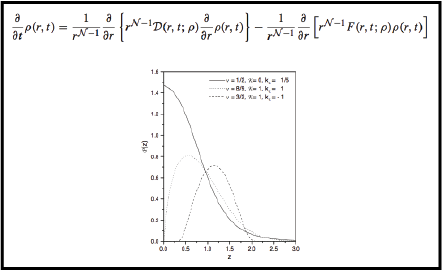
The solutions of a nonlinear diffusion equation by considering the radially symmetric N-dimensional case are investigated. This equation has the nonlinearity present in the diffusive term and external force. The solutions are obtained by using a similarity method and connected to the q-exponential and q-logarithmic functions which emerge from the Tsallis formalism. In addition, the results obtained here may be useful to investigate a rich class of situations related to anomalous diffusion.
Type