Fractional diffusion equations coupled by reaction terms

Abstract

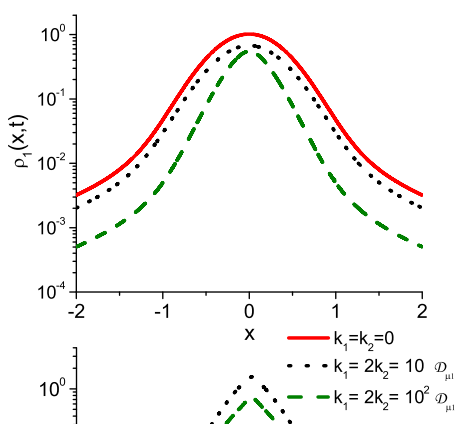
We investigate the behavior for a set of fractional reaction–diffusion equations that extend the usual ones by the presence of spatial fractional derivatives of distributed order in the diffusive term. These equations are coupled via the reaction terms which may represent reversible or irreversible processes. For these equations, we find exact solutions and show that the spreading of the distributions is asymptotically governed by the same the long-tailed distribution. Furthermore, we observe that the coupling introduced by reaction terms creates an interplay between different diffusive regimes leading us to a rich class of behaviors related to anomalous diffusion.