Abstract
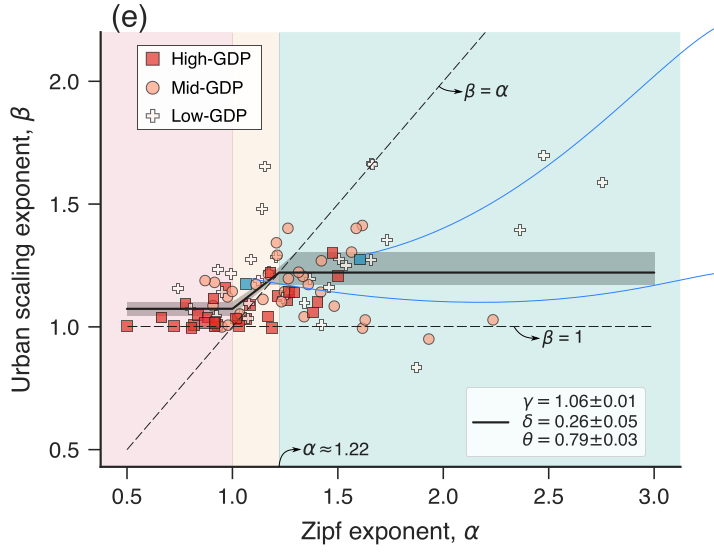
This chapter revisits two approaches connecting Zipf’s law and urban scaling – two of the best-known examples of regularities emerging in urban systems. The first approach shows that when an urban indicator is asymptotically distributed as a power-law function, the urban scaling of that indicator can be predicted from Zipf’s law of city population and its probability distribution. In its turn, the second approach demonstrates that the combination of Zipf’s and the urban scaling laws leads to country scaling relationships, and while the Zipf and the urban scaling exponents vary from country to country, there is only one value for each country scaling exponent, which in turn implies a direct association between the urban scaling and the Zipf exponents.
Haroldo Valentin Ribeiro
Associate Professor
Ervin Kaminski Lenzi
Associate Professor
